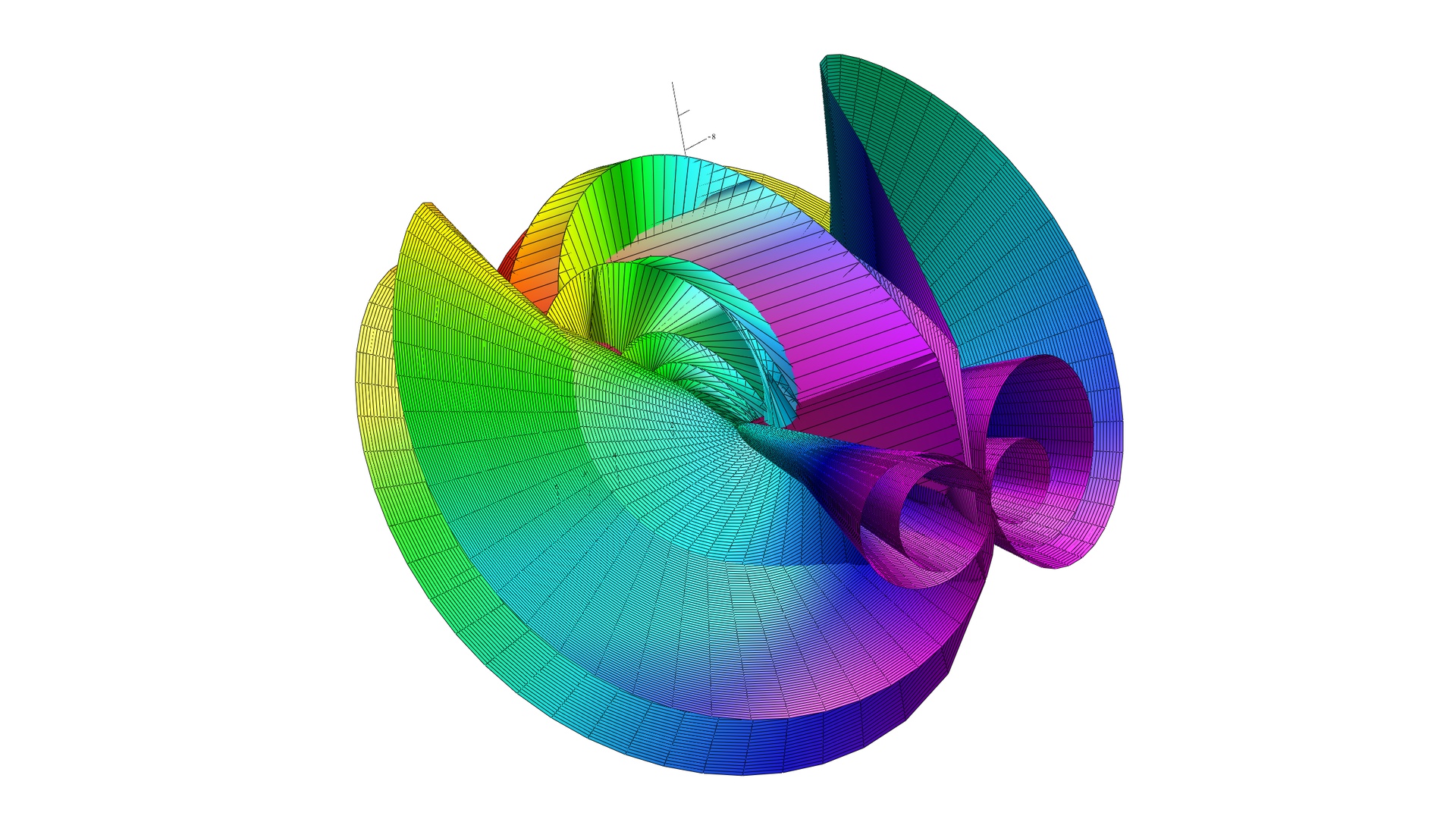
Freeness

xy³z+y⁵+z³=0

This surface is a representative of the species of "free" surfaces. Freeness is an algebraic property, which is not so easy to explain: roughly, it means that a free surface has singular curves, which have a very "nice" algebraic structure.

Free hypersurfaces were introduced 1980 by Kyoji Saito. Since then there has been done a lot of research revealing interesting properties of free divisors, and surprisingly free divisors pop up in quite different areas of mathematics, like deformation theory, differential geometry and combinatorics.

Author: Eleonore Faber

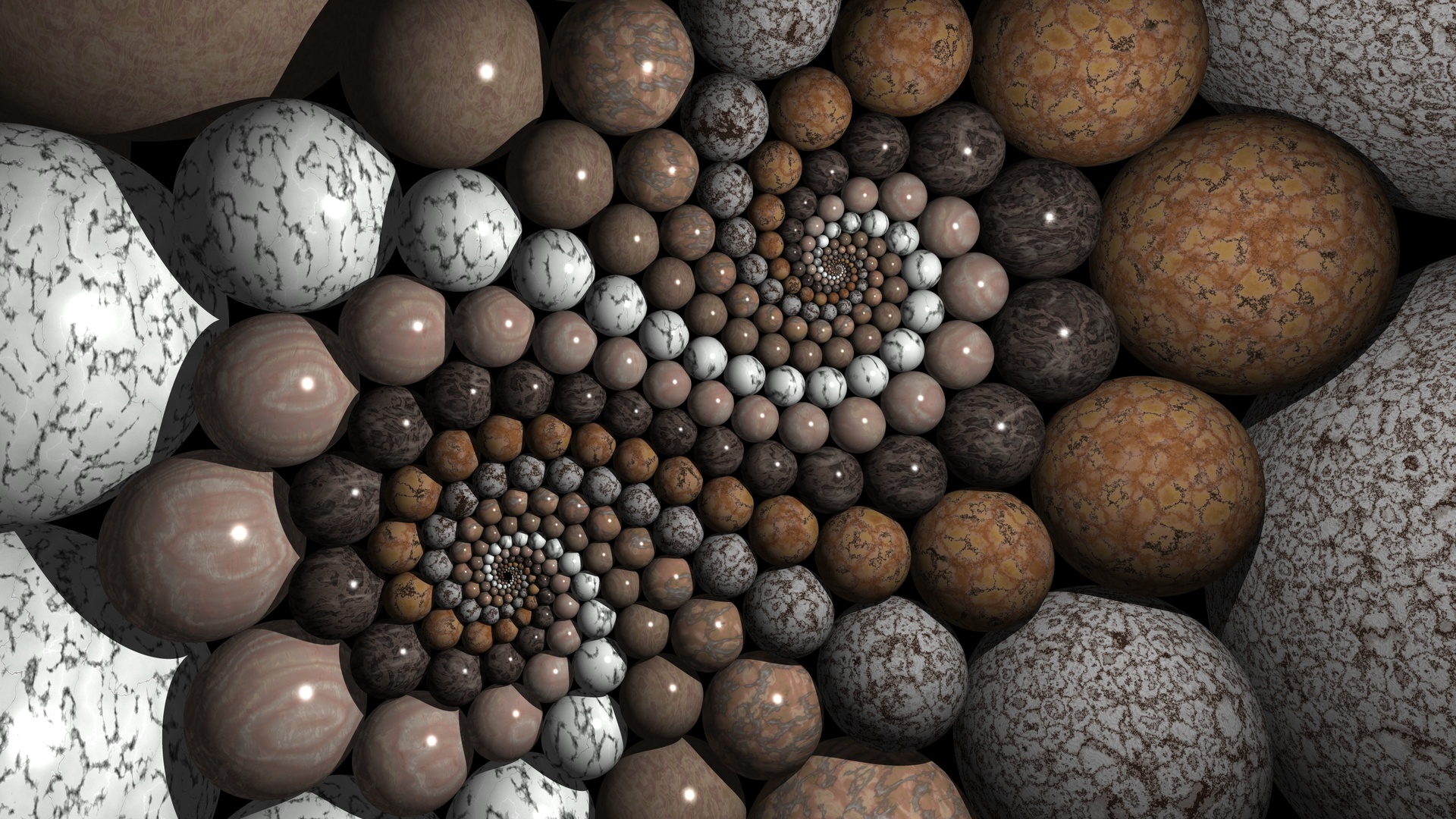
Circle Packing

Circle packing can be seen as the art of placing tangent circles on the plane, leaving as little unoccupied space as possible. The so called Apollonian Gasket recursively fills the space between tangent circles. But this method leaves large empty spaces inside the initial circles. The Steiner Chain method puts a chain of tangent circles between two circles. Here, two methods are combined and repeatedly applied.

Author: Francesco De Comité

Cyclotomic Creation

This image was generated through Maple Mathematics software. The author is basically sculpting with mathematics, using as his chisel trigonometric, group and number theoretic functions (and composed functions) so as to meticulously surf texture, scale and graphical behavior.

Author: Kenneth Neil Rubenstein

The Cardioid

The cardioid is a very current curve which can be generated in a lot of different ways: rolling a coin around another one, by reflection of sunlight into a milk pan (may be the first encounter with a not so simple mathematical object for children).

Pedoe method defines it as the envelop of a set of circles. If you rotate those circles in order to produce a 3-dimensional object, and if you try different ways to control this rotation, you can produce an infinity of very appealing structures, like this one, which inspired British milliner Gabriela Ligenza to create the first line of a 3D-printed hat.

Author: Francesco De Comité

Hamiltonian Circuit

It is known, that every archimedean solid has a hamiltonian circuit lying on all of its vertices. It is not so easy to find one of those circuits.

The author succeeded in finding a 104 vertices path on a truncated icosidodecahedron (instead of 120) and then connected those 104 points with a curved line, and afterwards duplicated this path five times, with the same transformations that produces the compound of five tetrahedra from a single initial. The structure was then rotated in order to hide the less symmetric parts.

Mathematics can be beautiful, even if not perfect.

Author: Francesco De Comité

Bohemian Stars

A collection of famous and/or interesting mathematical shapes and surfaces created with the software K3DSurf, which is a program to visualize and manipulate mathematical models in three, four, five and six dimensions. It supports parametric equations and isosurfaces.

Author: Torolf Sauermann

Lawson symmetric CMC surface

This is a cut-through view of a constant mean curvature surface (critical surface for the area with fixed enclosed volume) based on Lawson genus 2 minimal surface, analogous to a Delaunay torus.

It was recently discovered (2013) using Hitchin abelianization and the Dorfmeister-Pedit-Wu (DPW) Method.

Authors: Sebastian Heller, Nicholas Schmitt

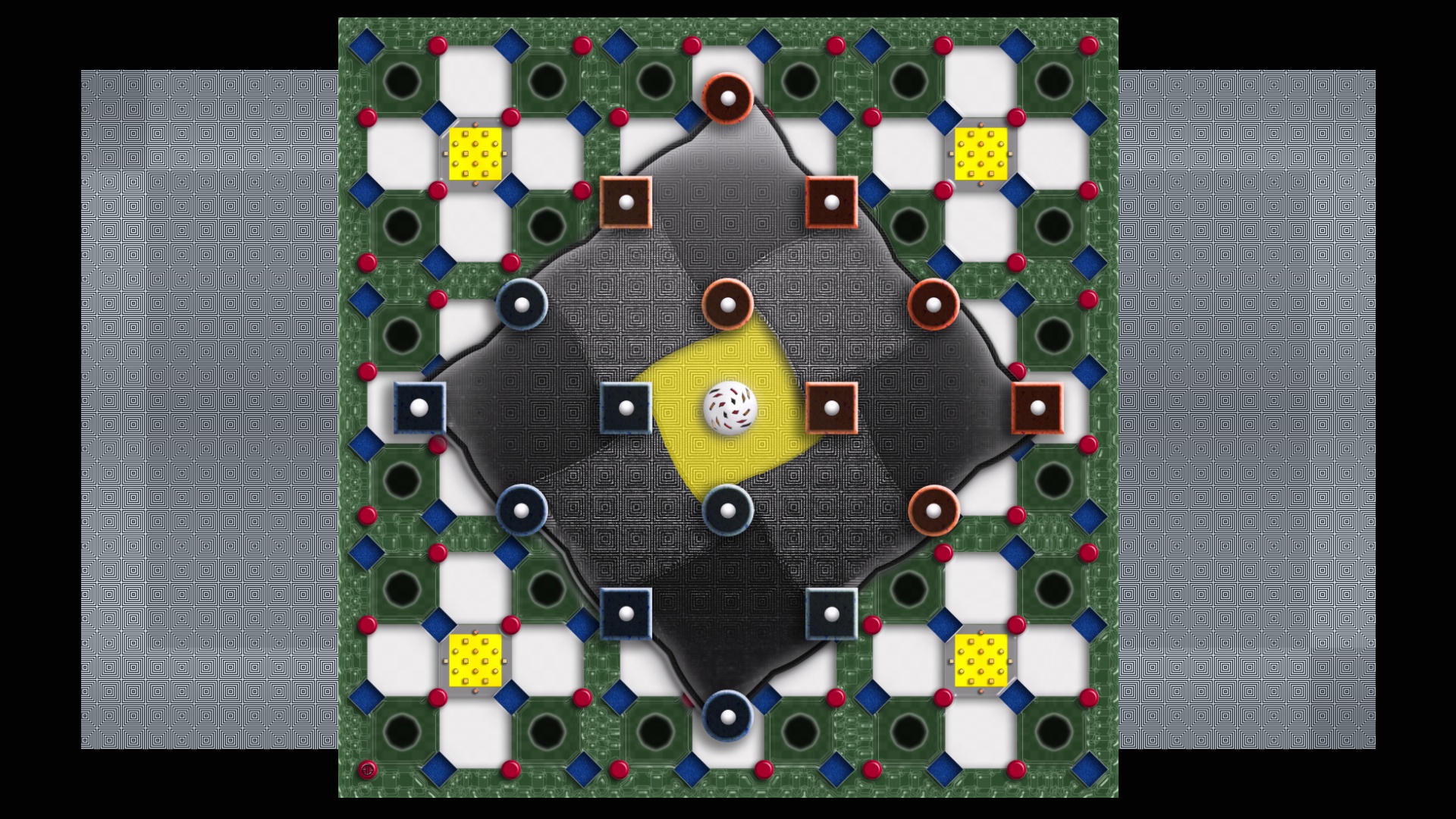
Classic Graph Theory Model 'The Shortest Route Problem'

The numerical reasoning needed to solve the 'Shortest Route Problem' has been emphasized by graphic cues of various shapes and color intended to guide the viewer thought the problem solving steps.

The specific color palette is based on the Korean traditional color palette - red, yellow and blue, plus black & white.

Author: Jean Constant

Isometric Symmetry

This is an illustration of one of the 32 crystallographic point group symmetries called isometric symmetry. A mathematical object is symmetric with respect to a given mathematical operation, if, when applied to the object, the operation preserves the property of the object. The isometric system is the most symmetrical system possible in three dimensional space.

The isometric unit cell is distinguished by four lines, called axes of threefold symmetry, about which the cell can be rotated by 120 degree without changing its appearance. Diamonds and red spinels belong to the isometric system.

Author: Jean Constant

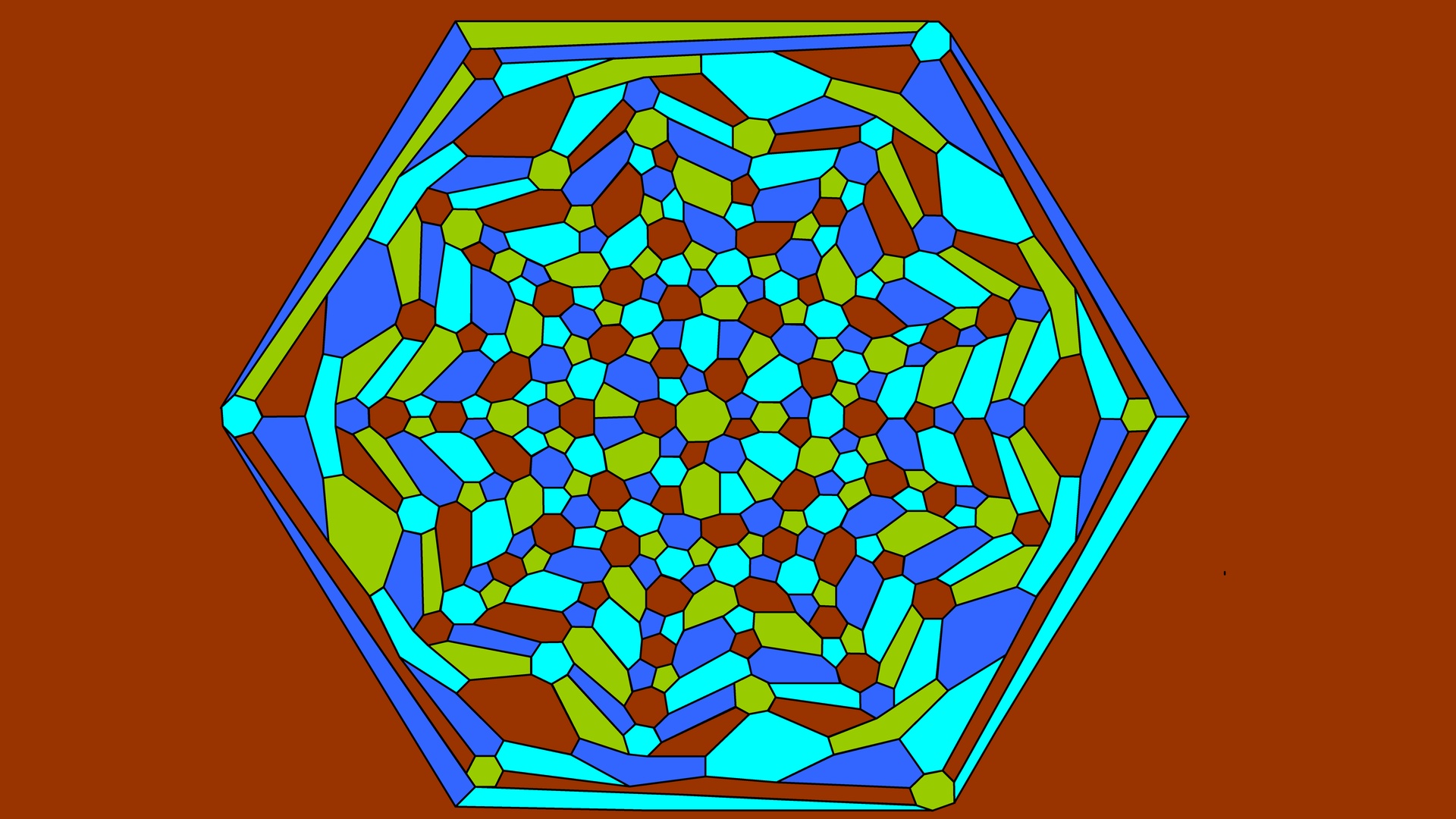
The Four Color Map

The four color map problem is to color regions of a map with at most four colors so that neighboring regions (countries) are presented in different colors. This simple problem was posed and conjectured to be true by Guthrie in 1852, although first proven in 1976 with the help of computers. The author presents the problem using only methods of the Victorian age in which the four color problem arose. The image illustrates the last of the three steps of the algorithmic proof.

Author: Ibrahim Cahit Arkut

Turtle Geometry

Turtle Geometry engages students in exploring mathematical properties visually via a simple programming language. This example shows a polygonal line consisting of a series of points connected by lines with unit length. The coordinates of each point are calculated iteratively following simple rules.

The result is a beautiful figure with sixfold symmetry. By modification of the parameters interesting figures of different shape and symmetry are obtained.

Author: Christian Gaier

Pixel Symphony

This image is generated by a simple program based on defining the color of each pixel using polar coordinates of the actual pixel combined with random values of constants which represent the genetic code of each program cycle.

At the beginning of each cycle the program defines a coloring palette, a random path through algorithms and a genetic code composed of 24 random values. Then each pixel is elaborated by going from the center towards the border. On every pixel a kind of mathematical "symphony" is performed, the result is the color of the actual pixel.

The program SYMMETRY08 is made by the picture author and can be downloaded at www.imaginary.org.

Author: Bogdan Soban

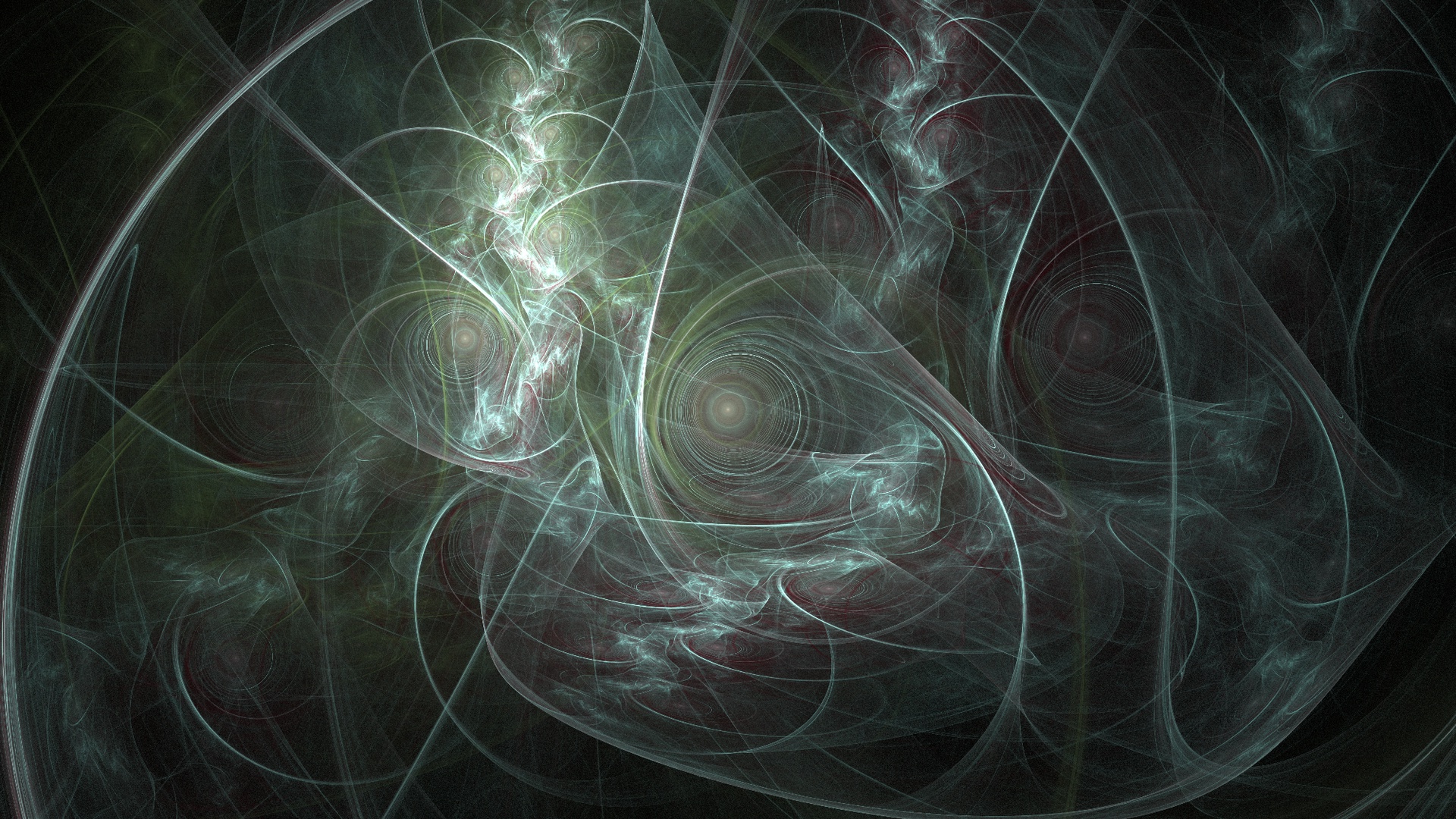
Apophysis

In 1988, Michael Barnsley developed the theory of Iterated Function Systems (IFS in short), a way of describing and generating fractals well suited for programming. The functions used were all affine transformations, and can generate objects like ferns, Sierpinski gaskets, etc.

Apophysis is a computer program, initiated by Scott Draves, which generalizes IFS by using non linear functions instead of affine ones. Its interface is quite complete and user friendly, allowing easy and fast experiments.

This picture was created using Apophysis. The program is a good illustration of what experimental mathematics can offer: fast tests and a large universe of possibilities.

Author: Francesco De Comité

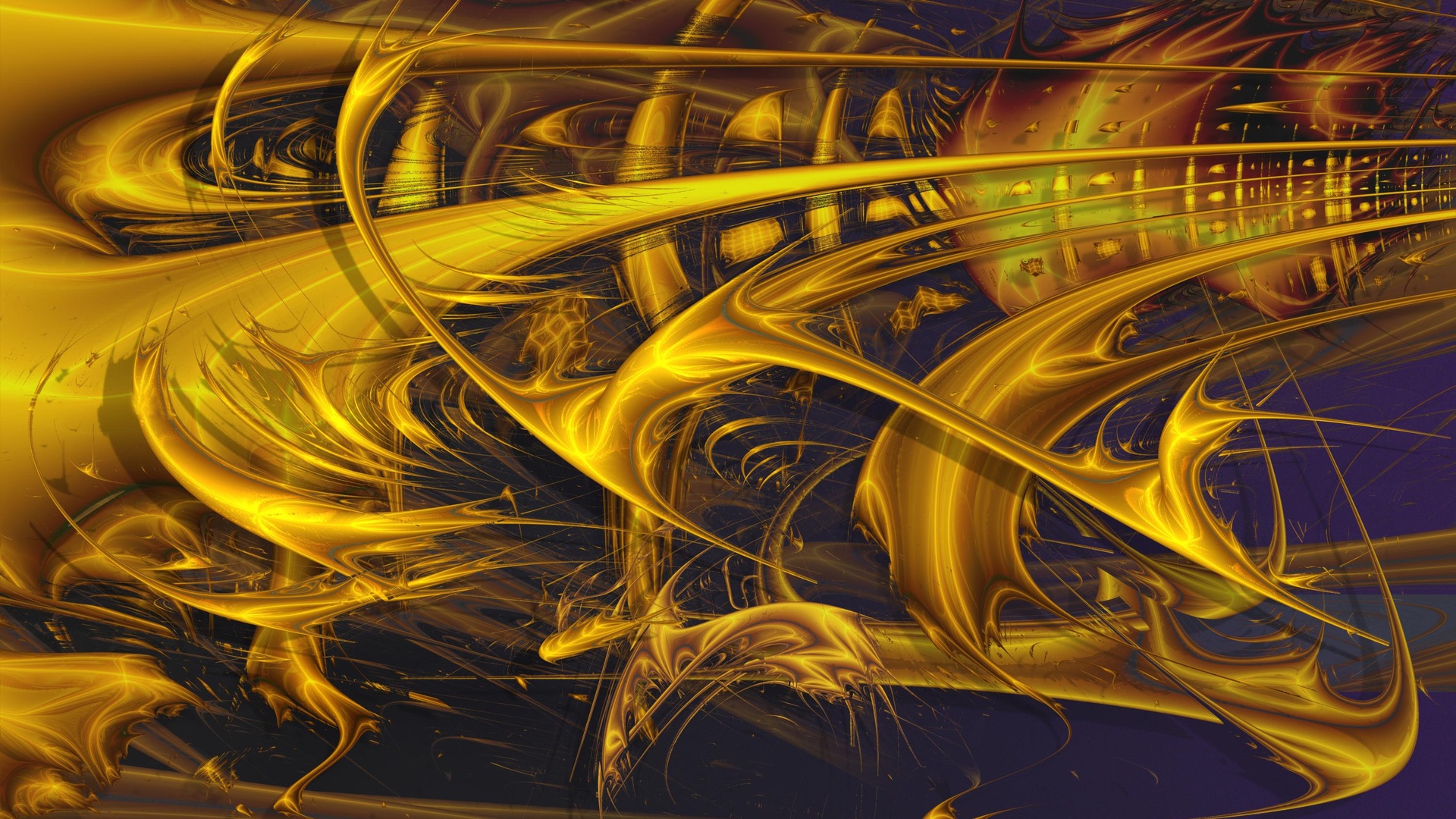
Lyapunov Play

Mario Markus of Max Planck Institute for Nutrition has used dynamical systems to study the evolution of animal populations - the change over time of food, fertility, size, etc. - with dynamics requiring the ability of reproduction to alternate quasi-periodically between two values. Such systems can show both a stable cycle and chaotic evolution depending on the fertility ability. Stability or chaos can be analysed by computing the so-called Lyapunov exponent. (Lyapunov was a Russian mathematician living at the end of the 19th century)

Markus-Lyapunov images are colour mappings of the Lyapunov exponent versus fertility, along horizontal and vertical axes. Only the stability domain is plotted; here, chaos (i.e. positive Lyapunov exponent) is rendered in dark blue. As the exponent goes from 0 to minus infinity, shades range from light to dark. At zero, the chaos threshold, the colour suddenly jumps from dark blue to a lighter shade. There is clearly much that is arbitrary in this colour mapping, and this gives an opportunity for choices based on aesthetic considerations. The picture consists of seven original Markus-Lyapunov pictures which were rebuilt and superimposed.

Author: Luc Benard

Transforming Cube

Slinky, the metal spring toy that walks down stairs, has been the key inspiration of the author. When squeezed, the transforming cube forms a cylinder with an ellipse as cross section. The endings remain circular, parallel or anti-parallel. The parts are assembled to make flexible structures. They led to the discovery of several transforming polyhedra.

Author: Xavier De Clippeleir

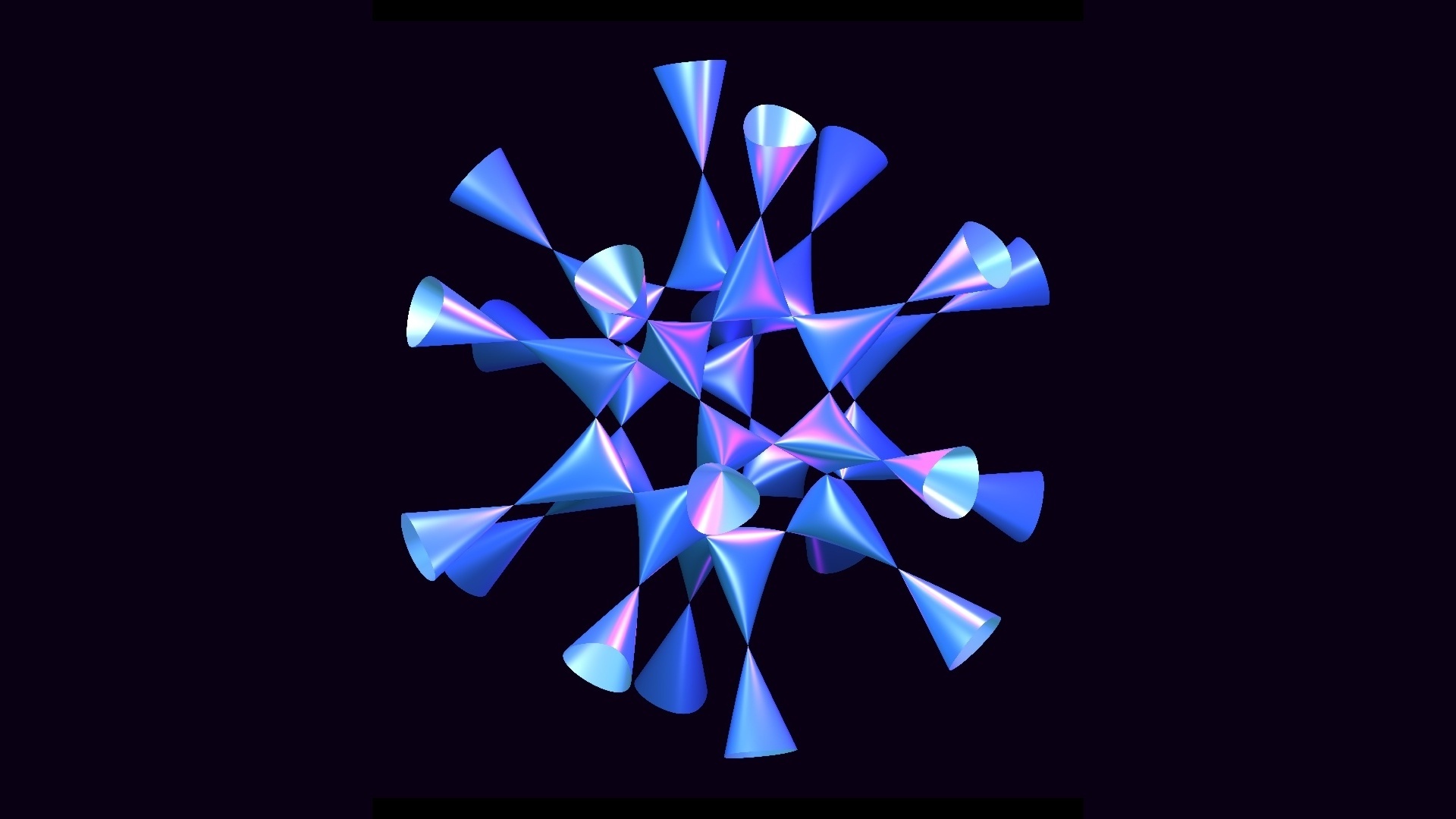
Barth Sextic

This surface of degree 6 (sextic) was constructed by Wolf Barth in 1996. Altogether, it has 65 singularities when also counting the 15 invisible ones which are infinitely far away. 65 is the maximum possible number of singularities on a sextic as shown in 1997 by Jaffe and Ruberman.

Barth's construction was a big surprise: For a long time geometers believed that a surface of degree 6 cannot have more than 64 singularities.

Author: Oliver Labs

Labs Septic

In 2004, while writing his Ph.D. thesis at Mainz, Oliver Labs constructed a surface of degree 7 (septic) with 99 singularities. Since 1997 one knows for surfaces of degree 6 that the maximum possible number of singularities is 65. In degree 7 there is a result of A.N. Varchenko from 1982 which tells us that there cannot be more than 104 singularities. In 1992, Chmutov constructed a septic with 93 singularities which was the world record at that time. Since Labs' construction, this world record has been 99. It is open if septics can have 100, 101, ... , 104 singularities.

The Labs Septic has the symmetry of a regular 7-gon. However, similar to Duco van Straten's computation for the sextics, one can compute that there is indeed a 5-parameter family of septics with 99 singularities. For the construction of the septic the computer algebra software Singular was used. It is developed at TU Kaiserslautern and very well suited for working with singular algebraic surfaces.

Author: Oliver Labs

Doyle Spiral

Doyle Spirals are a particular case of circle packing: each circle is surrounded with 6 tangent circles. When the parameters are well chosen (and this is the hard stuff), this circle packing can tile the plane. Applying to this tiling geometric transformations preserving the tangency property, one can create elegant patterns.

This work was initiated while looking at the beautiful and challenging images created by Jos Leys.

Author: Francesco De Comité

Boy Surface

The Boy surface is generated by adding a moebius strip with a disk attached to its boundaries to a closed surface. The fact that this is made possible at all was proved by Werner Boy. The Boy surface intersects itself, but otherwise looks smooth in each of its points.

The version shown here is characterized by its mean curvature being as small as possible. It has 'no unnecessary bumps' in this sense. Here, you see the most 'beautiful' possible realization of Boy surface in a mathematically precise sense. This is a parametrization of Boy surface by Robert Bryant and Robert Kusner.

The image is based on a bullet panorama which Paul Debevec generated from photos of a church in San Francisco. The scene was compiled in jReality, the image itself was calculated with Sunflow. Promoted by the DFG-Research Centre Matheon.

Author: Ulrich Pinkall

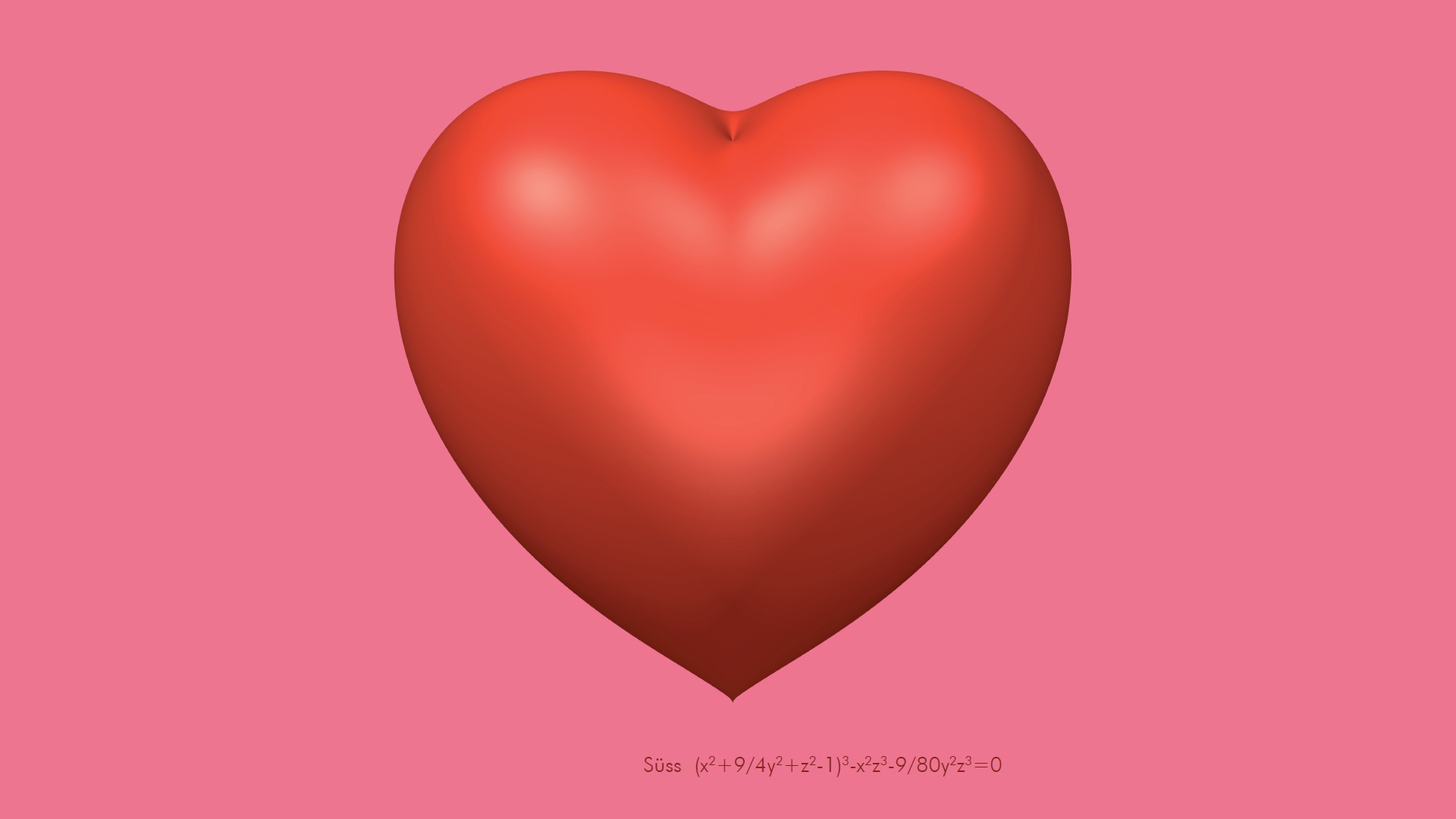
Süss

Love-letter

It can't go on and on like this!
This what we have we have to keep.
When we'll meet,
Something must be.
Together singularity.
What`s up on singularity?
For sure no platitudes.
Because what's singularity,
Is not wearing down so easily.
On a park bench in Grunewald
In two to face the rain,
Attempts in vain. Girl, take heart! Write me a card!
Yours, Bertie! (Erinner Singularity).

Poem by Joachim Ringelnatz

The passion of love is generally connected to the emotional power of a ''singularity''. This connection appears in many forms of art.

Author: Herwig Hauser

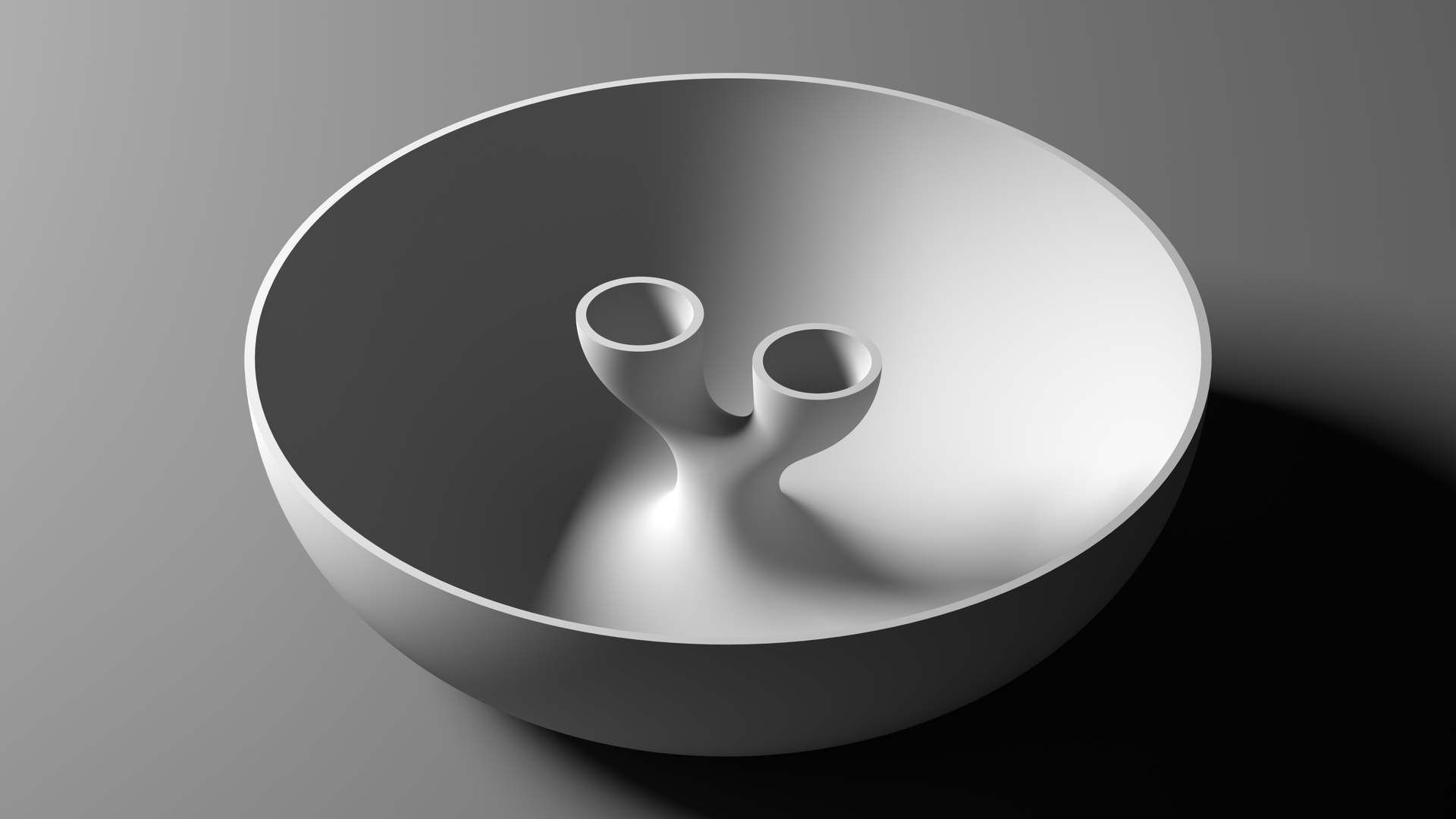
A Triply-Periodic Level Surface

The green-tinted surface pictured here has three orthogonal translational symmetries. It is the level-surface given by the trigonometric equation:

4 (cosx cos y + cosy cosz + cosz cosx) - 3 cosx cosy cosz = -2.4

A unit cell may be viewed as a central chamber with tubes to the corners and faces of the cube. This surface closely approximates the minimal P-Surface discovered in 1880 by Karl Hermann Amandus Schwartz (who was a Professor at Halle, Goettingen, and Berlin). Recently it has been investigated by material scientists, who use it and related surfaces to model so-called block- copolymers. The original model was taken from David A. Hoffman. This was textured, placed in a scene that we feel adds to the beauty of the object and rendered in Bryce.

Author: Luc Benard

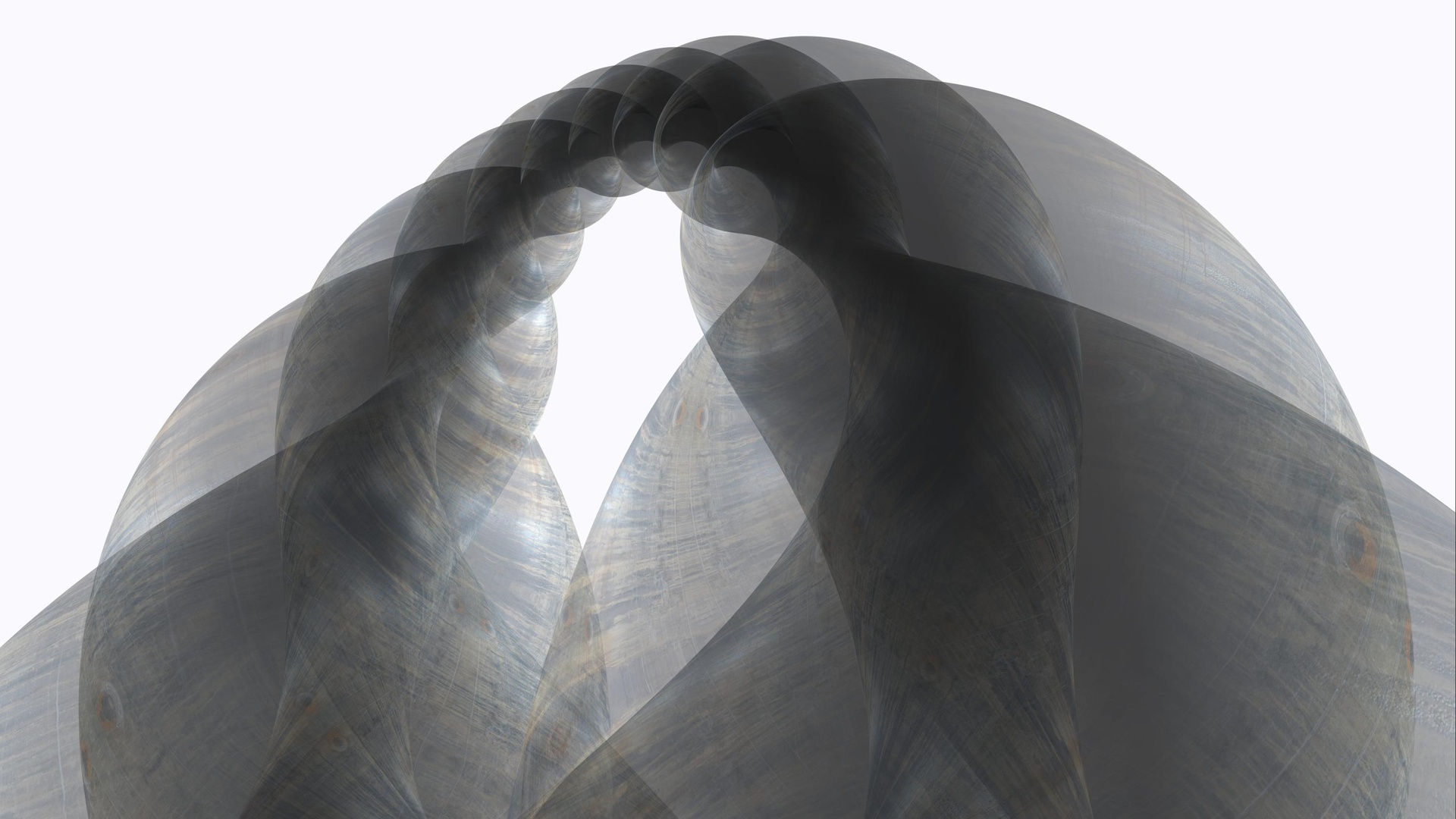
Twizzle Torus

The Twizzle Torus is an annular surface with a constant mean curvature in the three-dimensional sphere, a space curved in itself. To make it visible it must first be projected into our flat space. Luckily, this is enabled such that basic shape features can be maintained. In the three-dimensional sphere where it belongs to it has a screw symmetry which can still be surmised during projection.

The Twizzle Torus is only a comparatively simple example in an endless hierarchy of increasingly complex annular surfaces with similar curvature features.

The surface was developed by the GeometrieWerkstatt Tübingen using XLab software.

Author: Nicholas Schmitt

Suzhi Kolam

Kolams are decorative geometrical patterns that adorn the entrances of households and places of worship especially in South India (Tamil Nadu). It is a belief that laying kolam brings wealth and prosperity to home, business, etc. On Scientific basis, laying kolam is a good exercise for body, fingers and mind. It improves arithmetic knowledge and imagination power. While joining dots we concentrate our mind and it improves the strength of thinking power.

Dots (called pulli) are arranged in rhombic, square, triangular, or free shapes, and a single, uninterrupted linear or curvilinear line (called the kambi), intertwines the dots. There are no written or verbally stated rules.

Kolam drawing can be treated as a special kind of graph with the crossings considered as vertices and the parts of the kambi between vertices treated as edges. The only restriction is that unlike in a graph, these edges cannot be freely drawn as there is a specific way of drawing the kolam. The single kambi kolam will then be an Eulerian graph with the drawing starting and ending in the same vertex and passing through every edge of the graph only once.

Author: Brunda Alagarsamy